
Imagine holding a strip of paper. You give it a half-twist and then tape its ends together. The shape you’re now holding is the ticket to a world where surfaces have only one side and boundaries blur between inside and out. This is the realm of the Möbius Strip.
The Möbius Strip is one of the most intriguing structures we’ve encountered, a perfect blend of an ordinary shape with highly complex properties that has captivated mathematicians for over a century. One of the most challenging puzzles is a deceptively simple question: How tiny can a Möbius Strip get before it tangles within itself?
Back in 1977, mathematicians Charles Weaver and Benjamin Halpern first dropped this brainteaser into the academic world — and mathematicians have been left frustrated ever since, trying to find the right answer. Now, Richard Schwartz, a mathematician from Brown University, claims he has finally solved the puzzle.
When a circle isn’t a circle anymore
The Möbius Strip has a “non-orientable” surface. In everyday terms, this means if you were an ant crawling on its surface, you wouldn’t be able to distinguish one side from another. If you take a pencil and draw a line along the center of the strip, you’ll notice that the line runs along both sides of the loop. It’s quite mind-bending to see.
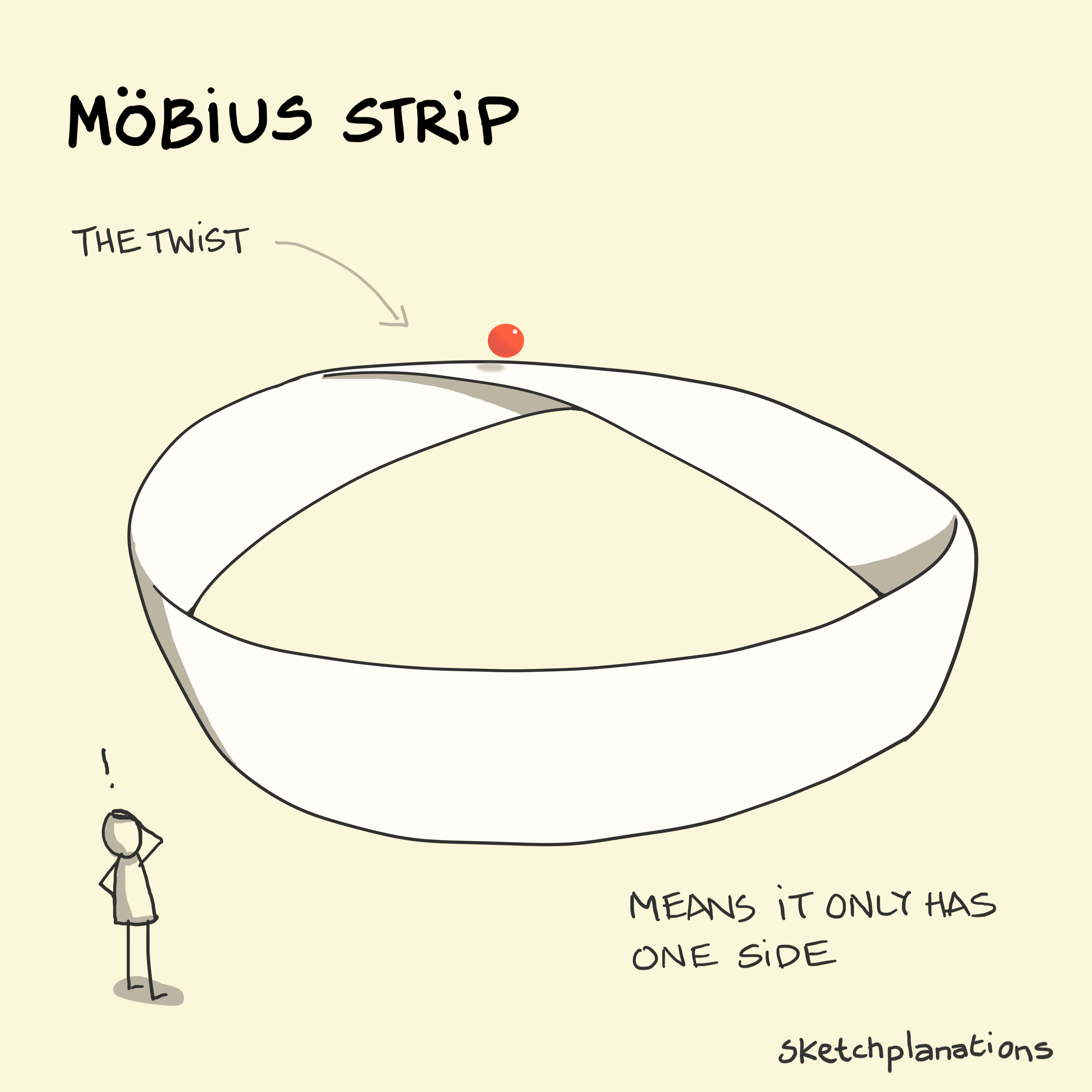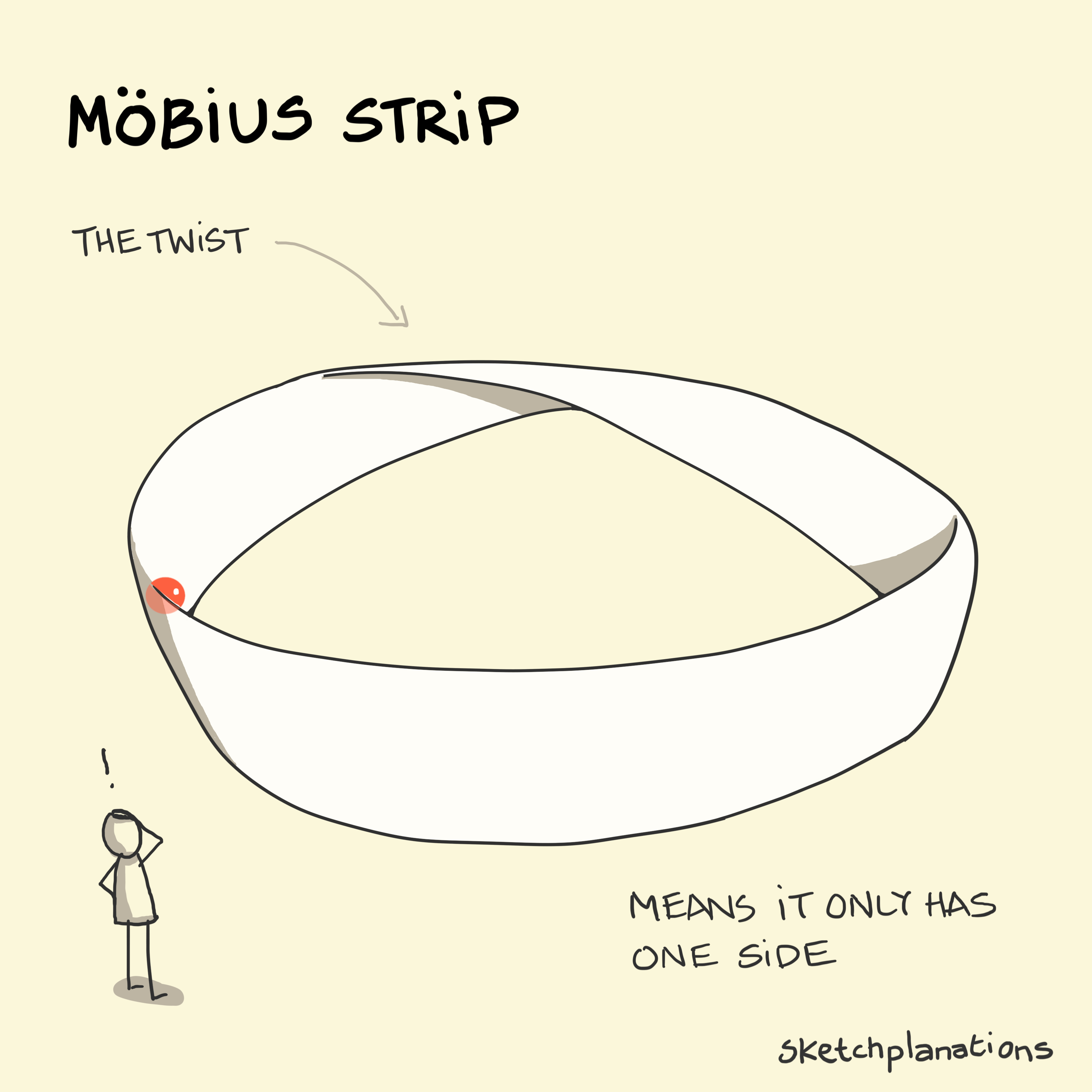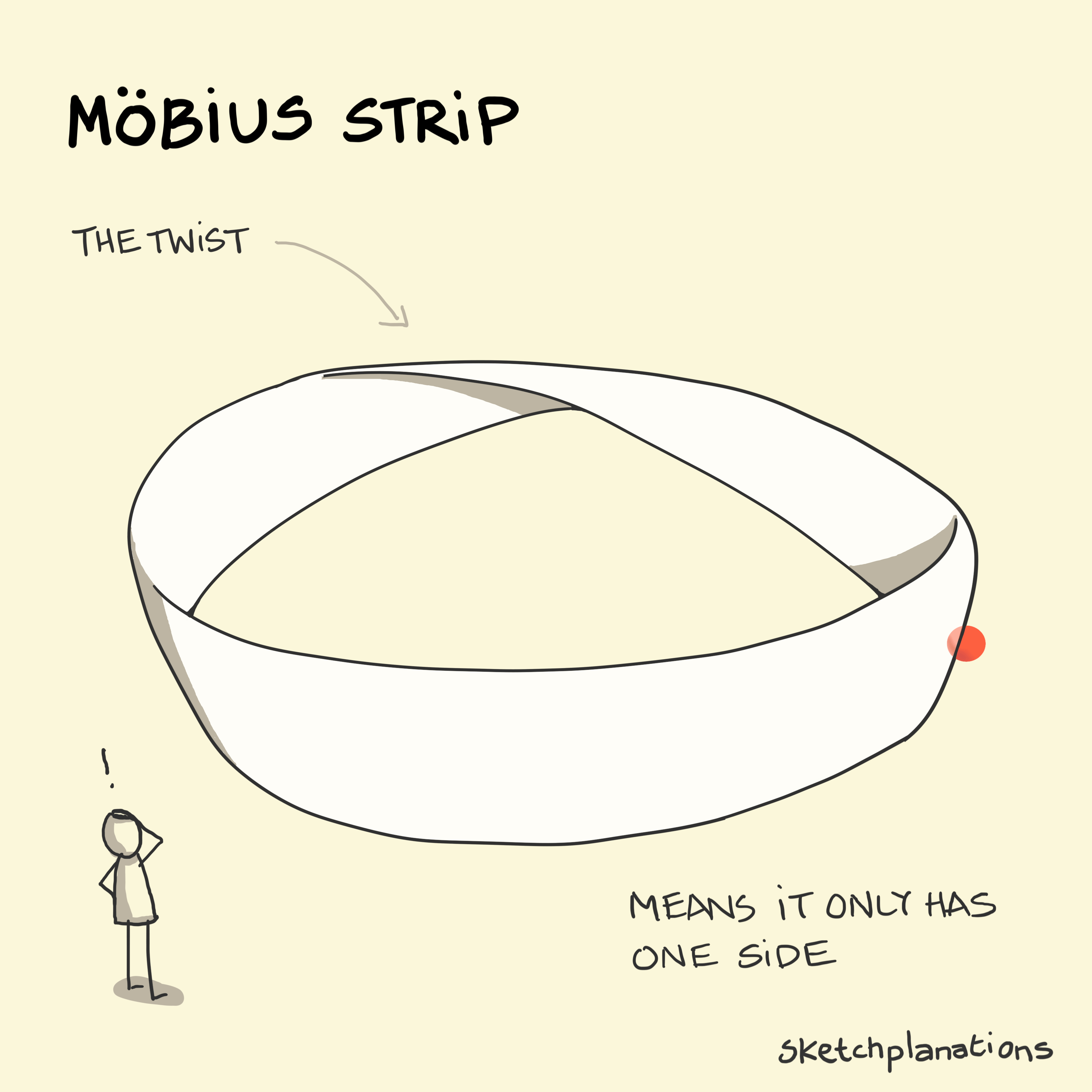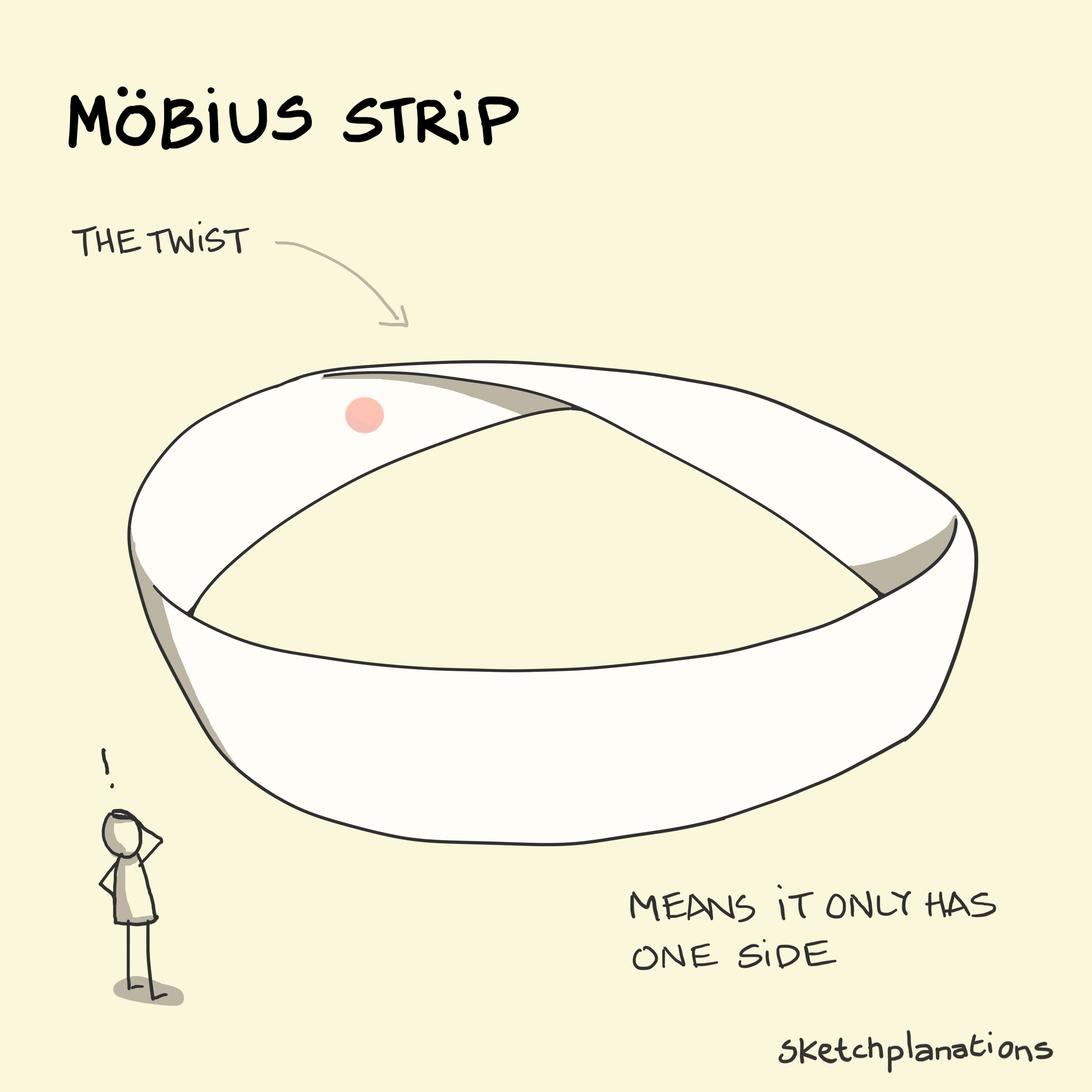
The German mathematicians August Ferdinand Möbius and Johann Benedict Listing independently discovered it in 1858. While Möbius got the naming rights, both men were drawn to its peculiar property: its unending surface.
This isn’t just some mathematical gimmick. Many engineers and scientists find the Möbius Strip fascinating for practical reasons. For instance, conveyor belts designed as a Möbius strip distribute wear and tear uniformly, lasting twice as long as conventional conveyor belts. In electronics, Möbius resistors are employed due to their unique electromagnetic properties.
Artists aren’t immune to the strip’s allure. M.C. Escher, the famed graphic artist, incorporated the Möbius Strip in his woodcut “Möbius Strip II,” where ants interlock and traverse the one-sided surface. Even the ubiquitous recycling symbol, found printed on the backs of aluminum cans and plastic bottles, is essentially a Möbius strip.
While the visual appeal of the strip is undeniable, its most significant impact has been in mathematics. Among its many contributions, the introduction of the Möbius Strip has revolutionized the field of topology, which studies the properties of objects that are preserved when moved, bent, stretched or twisted, without cutting or gluing parts together. A coffee mug and a doughnut are, for instance, topologically identical. Both objects have just one hole, which can be deformed through stretching and bending to create one or the other structure.

A breakthrough moment
But it’s not topology that intrigued Schwartz. He first heard about the minimum Möbius strip problem four years ago and has been hooked ever since. His efforts to untangle the Halpern-Weaver conjecture finally paid off. The mathematician reported the solution on the preprint server arXiv.org in August 2023.
His findings? The perfect Möbius strip should possess an aspect ratio greater than √3 (approximately 1.73). In layman’s terms, a strip that is 1 centimeter long must exceed 1.73 centimeters in width otherwise the structure will collapse.
Yet, the path to discovery wasn’t a straight line. Schwartz sliced, examined, and even flattened Möbius strips in his quest. As he grappled with the problem, he employed various strategies over the years. But an ‘Aha!’ moment arrived when he revisited a past error — a minor misidentification of a shape — and everything clicked from there.
“The corrected calculation gave me the number that was the conjecture,” Schwartz told Scientific American. “I was gobsmacked… I spent, like, the next three days hardly sleeping, just writing this thing up.”
However, as is often the case in mathematics, solving a problem opens the door to solving another, more complex one. There is no limit, mathematically speaking, to how long a Möbius strip can be. But the next problem on Schwartz’s mind is finding the shortest strip of paper that can be used to make a Möbius strip with three twists instead of just one.
Mathematics often pushes the boundaries of our understanding, nudging us to question the very fabric of reality. And in this fabric, the Möbius strip stands out as a mesmerizing thread, reminding us of the beauty that lies in endlessness and continuity.


