Each sport has its own version of the curveball, a trajectory that bends in the opposite direction of the ball. It only happens when the ball spins and moves forward at the same time, a phenomenon called the Magnus effect. But the Magnus effect is a bit of a physical curveball itself — one that relies on the physics of turbulence to work its magic.
Bernoulli’s principle
Air and water are both fluids, which means they have the ability to flow around various objects and allow objects to move through them. Fluids flow in distinct paths called streamlines, which represent the direction and shape of the fluid as it moves around an object. Researchers have conducted extensive studies to understand fluid flow, and one of the key concepts that emerged from this research is the Bernoulli principle.
The Bernoulli principle
Bernoulli’s principle is a mathematical relationship between the pressure and velocity of a fluid. It only applies when the fluid’s properties remain constant, like in the case of calm water. To better understand this principle, you can perform a simple experiment with a sheet of paper. Hold the paper by its edges with your fingers, allowing the majority of the sheet to hang freely. Gently blow air over the top of the paper, and you will observe the paper rising and remaining straight as long as you continue to blow air over it. This phenomenon occurs because the air pressure beneath the paper is higher and the air is relatively still, while the air above the paper moves with greater velocity and has lower pressure.

When an object has a curved shape, it can effectively follow the contours of streamlines. This causes a pressure gradient, which in turn generates a force that redirects the object. For instance, an airplane’s wings have airfoils that are curved on the top. As a result, the pressure gradient produces a lifting force that helps the airplane take off and maintain altitude.
Bernoulli’s principle can be applied to various types of fluid flow, and it can be seemingly counterintuitive, but the core idea is that within a horizontal flow of fluid, points of higher fluid speed will have less pressure than points of slower fluid speed.
Bernoulli’s principle is applicable to a variety of fluid flow scenarios. Although it may seem counterintuitive at first, its central concept is that within a horizontal flow of fluid, areas with faster fluid velocity will have lower pressure compared to areas with slower fluid velocity. This principle helps explain various fluid dynamics phenomena in nature and engineering. It also ties into our core attraction: the Magnus Effect.
Boundary layer
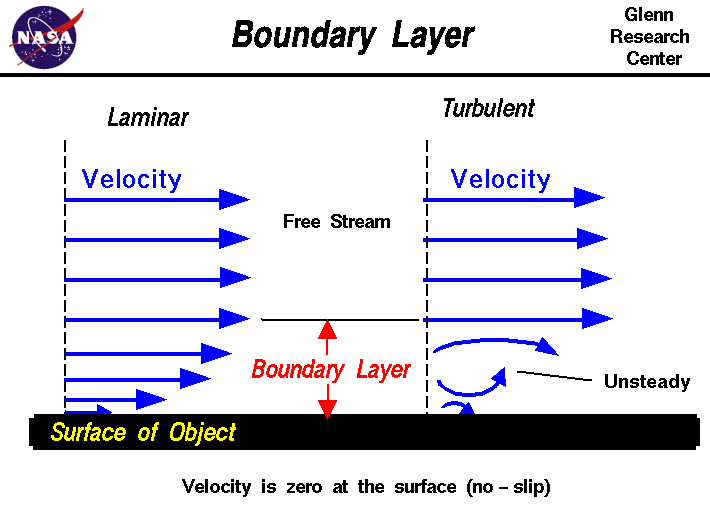
Whenever a shape is immersed in a fluid, there is a thin layer surrounding it called a boundary layer. It divides the flow and the air slows down near the object’s surface.
The boundary layer can be laminar when the flow is very calm like adjusting the flow of a tap to a minimum pressure. Or it can be a turbulent boundary layer when the speed of the fluid is high, so the water of the tap falls chaotically and there are drops out of the flow straight path.
When the flow of fluid is very gentle (such as a faucet with minimal water pressure) the boundary layer can be laminar. This means it flows smoothly and consistently. On the other hand, when the fluid’s speed is high, the boundary layer can become turbulent. In this case, the water from the faucet falls erratically, and droplets may deviate from the main flow’s straight path. This is where the Magnus effect starts to take shape.
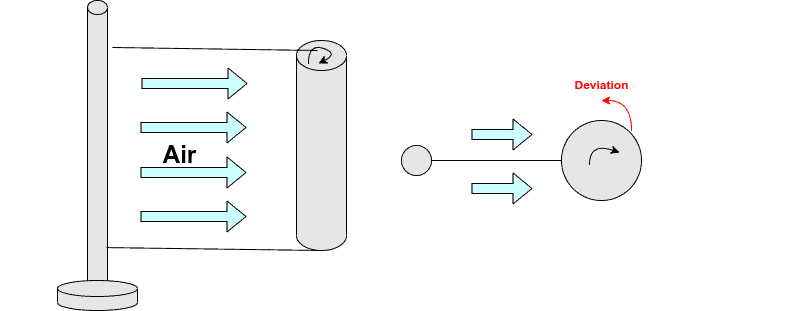
Magnus force
For a circular object rotating around its axis, the fluid flow can significantly alter its path. Gustav Magnus explained the idea through an experiment in 1892. He put a cylinder attached to its axis and directed a current of air toward it. At the same time as the cylinder spins the current redirects the object in a trajectory opposite to its own spin. The phenomenon is the Magnus effect.
A clockwise spinning cylinder is pushed in the opposite direction, like in the figure, it goes up. Conversely, a counterclockwise movement would push the cylinder down. This push is conducted by the Magnus force.
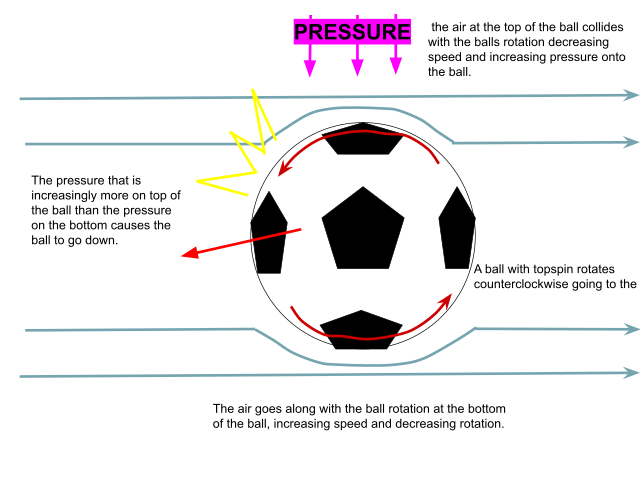
There is a pressure gradient that forms this force, but it is not the same as Bernoulli’s principle. In this case, turbulence is relevant to the phenomenon. In the paper experiment, as the air flows around the paper in a laminar flow, there is no way to form regions where turbulence is significant. For cylinders or spheres, the space behind the air flow forms small vortices in the turbulent boundary layer.
The asymmetry in the boundary layer is the cause of the pressure difference. The pressure is lower where the vortices form, making the flow right below the ball and forcing it to bend its path.
The Banana shot
The Magnus effect shows up in real life quite a bit. It even shows up in sports, believe it or not.
Skilled football players use the Magnus effect in their favor to curve free kicks. This is useful when there is a wall of players in front of the goal. The ball will circumvent the wall and reach the goal.
In 1997, Roberto Carlos, a former Brazilian football player, performed this kick in a match against France. The goal was nicknamed the “Banana shot” due to its curved shape. The speed is crucial otherwise there would be no turbulent flow formed in the ball’s boundary layer. The shot’s speed was over 100 kilometers per hour (62.14 miles per hour).
Besides speed, the time spent interacting with the air so that the turbulent layer forms is also important. The ball was around 35 meters away from the goal. If the freekick spot was too close, the ball wouldn’t be pushed by the Magnus force. If it was too far away the kick wouldn’t be strong enough to reach the goal. The distance needed to be just right — and it was.
But the distance is not enough. You also have to kick the ball the right way. This means kicking the ball with the outside part of the foot, which helped the ball rotate counterclockwise. Using physics and the Magnus effect, Roberto Carlos scored one of the greatest goals in history.
The Magnus effect often pops up in sports that involve spinning balls, like football, golf, basketball, tennis, baseball, volleyball, table tennis, and cricket. However, this effect plays a role in more than sports. For instance, it can be significant in ballistics, and, of course, in aviation and sailing. Some aircraft have been built to use the Magnus effect to create lift with a rotating cylinder instead of a wing, but the design didn’t prove successful.
Ultimately, the Magnus effect is a fascinating concept in the field of fluid dynamics, with far-reaching implications in various aspects of our lives. From sports such as soccer and tennis, where players manipulate the spin of the ball to create unexpected trajectories, to the aerospace industry, where engineers apply the Magnus effect to design advanced airfoils and winglets, this phenomenon continues to play an important role in our understanding of fluid mechanics. After all, it’s not every day that an effect pops up in soccer and ballistics.